Single-Crystal versus Powder Diffraction

Course Material Index  Section Index  Previous Page Next Page

Single-Crystal versus Powder Diffraction

Laue Classes

In a single-crystal diffraction experiment, the intensity of the spots is measured as a function of the Miller indices hkl. When the space-group symmetry is unknown, reflections can be measured and compared with other possible symmetry equivalent reflections.

For example, in a particular experiment the unit-cell geometry may be such that a ≠ b ≠ c and α = β = γ = 90° within experimental error. Typically the esd's on the angles will be relatively large and so to test for orthorhombic symmetry it is necessary to check, say, that I(hkl) = I(h-k-l) and I(hkl) = I(-h-kl) to demonstrate that twofold symmetry with respect to a* and c*, respectively. This is often referred to as a Laue check on the symmetry of the crystal. Most single-crystal software programs carry out this sort of check automatically and thus deduce the correct Laue class for the crystal.

In powder diffraction, all the symmetry-equivalent reflections have the same d spacing with the result that individual intensities cannot be measured. Hence, there is no equivalent test to the Laue check of the single-crystal experiment. The unit-cell geometry is normally determined very precisely, which helps, but does not solve the problem. The situation is made worse in the higher-symmetry crystal systems since the cubic, tetragonal, hexagonal, and trigonal systems all possess more than one Laue class. The worst case for the determination of Laue class in powder diffraction is when the unit-cell geometry corresponds to a primitive hexagonal lattice since there are five possible Laue classes which are indistinguishable. This is summarized in the table below:

Reflection Conditions

Reflection conditions play a vital role in the determination of space-group symmetry. In a single-crystal experiment, where each reflection is measured individually, computer programs can automatically test for general reflection conditions due to centred lattices, plane reflection conditions due to glide planes, and line reflection conditions due to screw axes. In general, there are usually sufficient data that determination of the reflection conditions is not a problem.

Contrast this with a powder diffraction experiment, where peak overlap due to the presence of reflections with similar d spacings is the norm. Identification of the reflection conditions for a centred lattice is usually not too difficult as demonstrated by the stick diagrams below, which were generated for Cu Kα₁ and an orthorhombic unit cell with a = 6.0 Å, b = 7.5 Å, and c = 8.5 Å. The density of reflections is very different in each of the three examples shown below:

Primitive lattice e.g. Space Group P222

C-centred lattice

F-centred lattice

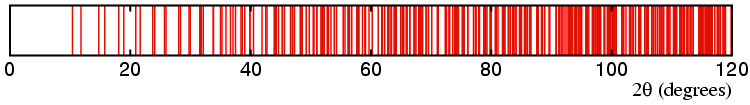
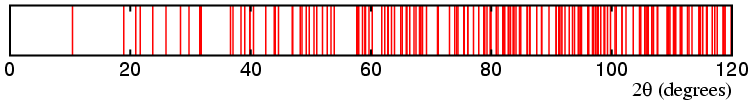
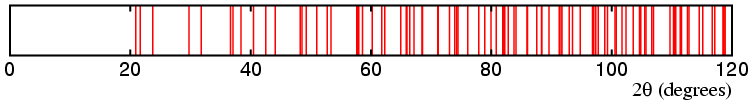
Using the program for generation, you can demonstrate this for yourself for P, C-, and F-centred lattices.

Now contrast this with the determination of a screw axis reflection condition as shown in the diagram below, which was generated assuming the space group to be P222₁:

Space Group P222₁

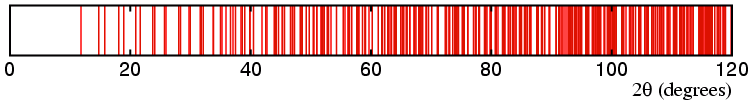
The only obvious difference between this pattern and the previous one for space group P222 is in the first reflection. Given that the first reflection might be very weak, it would then be difficult to determine whether the reflection condition 00l: l = 2n was present or not. Thus screw-axis reflection conditions are difficult to observe with any degree of certainty in powder diffraction data.

Reflection conditions due to glide planes usually fall somewhere between the two extremes demonstrated above. There is usually sufficient information in the powder diffraction data to identify the presence or absence of glide planes, especially when the lattice is primitive. This is discussed in more detail in the next page.

Reflection Intensity Statistics

There is another big difference in the analysis of single-crystal and powder diffraction data regarding the determination of space-group symmetry. The intensities measured in a single-crystal experiment can be converted to what are known as normalized structure factors or E values. The quantity <|E² - 1|> has a statistical expectation value of 0.968 if the crystal structure is centrosymmetric and 0.736 if not. <|E² - 1|> values are only indicative since in practice the experimental values often deviate from the expected statistical value.

The same quantity cannot be used at all reliably with most powder diffraction data due to the problem of a obtaining a sufficient number of reliable intensities I(hkl). This provides an added difficulty in the determination of space group symmetry from powder diffraction data since centrosymmetric and non-centrosymmetric space groups are often indistinguishable in terms of reflection conditions alone.

Course Material Index  Section Index  Previous Page Next Page